Problem:
You initially have one Amoeba. She can split into 0,1,2,3 all equally likely. What is the probability of the Amoeba population eventually dies out?
Solution:
This is a special case of a classic stochastic process known as a branching process. If you don't know about them, or feel rusty, feel free to book a session now and we're happy to each them to you !
I TeX'd up the solution because of the notation which you can find below
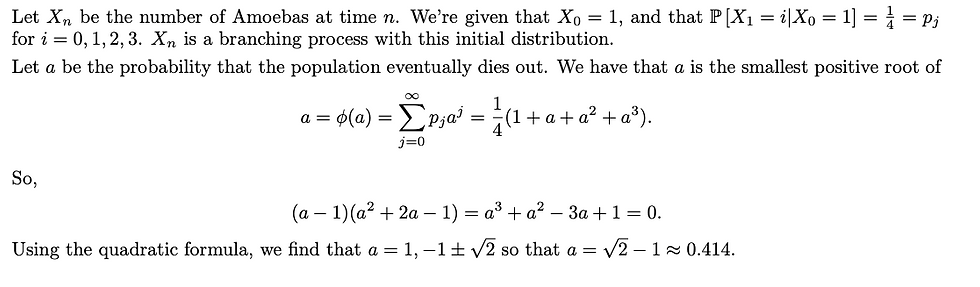
Candidates get as far as the setup, but sometimes have difficulty computing the actual probability.
The easiest way to remember how to do this is to remember that the probability that the population dies out is a steady state statement.
That means that the probability the population eventually dies out doesn't depend on time. In other words, a (now) must equal a (later).
If we increment the process once step into the future, what's the value of a?
Well, a single Amoeba might just die right then and there which occurs with probability,
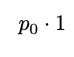
She could also just produce one offspring which then dies, which occurs with probability,

She could also produce two offspring, each of which must also die which occurs with probability
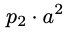
She could also produce three offspring, each of which must also die which occurs with probability
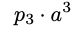
and so on...
Since these are mutually exclusive events, we can just sum them all up to get the desired probability.
Since the value of a now must equal the value of a at this later time, we arrive at the detailed balance equation,
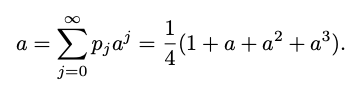
Feeling lost? Book your first session now to help clarify anything !
Why the smallest positive such root of this detailed balance equation?
Let's plot the RHS and LHS of the detailed balance equation in this problem to get some intuition:

We can see they intersect exactly twice, and that one of the roots is always 1. This makes sense, since a=1 is always a solution to the detailed balance equation.
In general - the picture always looks like this:
a=1 will always be a solution.
p(0)>0 forces the y-intercept of the RHS to be larger than the LHS in the detailed balance question.
The RHS of detailed balance is always convex. To see this, just take two derivatives of the RHS and notice it's always positive.
This means there's always either 1 or 2 solutions, with one always being a=1. In the former case, extinction is certain. In the latter case, extinction is uncertain and we're interested in the solution a<1.
Book your first session now to master Markov Chains and ace the Quant Finance Interviews !
Want more?
Learn how Roth IRAs and Mega Backdoor Roth IRAs could generate millions tax-free in your post-tax retirement strategies !
Estimate how much you could have in retirement using Pre-Tax Strategies that could reduce your taxes now and nab you millions at retirement.
Subscribe to our Substack page to read and keep up-to-date on upcoming strategies.

Comments